Models, Functions, and Graphs

Construct appropriate functions for each of 1-7, below, and use Graphing Calculator to estimate the question's answer.

Construct complicated functions in pieces, so that each piece is a building block in constructing a representation of the situation. Use GC's text feature to annotate each function.

You can estimate a value graphically by clicking on a place on a graph, then reading the coordinates of the mouse click (and the value of any evaluated expressions) in upper left corner of the graph window.

Do this for each of 1-7:

Draw a labeled diagram of the situation
List the functions that describes how quantities covary.
For each function defintion, state what each variable and expression represents about the situation.
Explain what the behavior of the function's graph shows you about dynamic aspects of the situation.

Example:

It is 1873 in Territorial New Mexico. The Lone Ranger is chasing a desparate bank robber over the desert. Both are on horseback, and the bank robber got an 11 mile head start. The bank robber's horse can run steadily at 16 miles per hour. The Lone Ranger's horse can run steadily at 19 miles per hour. The bank robber is heading for the Mexican border, for if he can cross the border he will be safe. The town that they started from is 49 miles from the border. Will the Lone Ranger catch the bank robber?

x = number of hours that have passed since LR began chasing the bandit.

f(x) = 19x This is the Lone Ranger's distance from town x hours after he started chasing the bank robber

g(x) = 16x + 11 This is the robber's distance from town x hours after the Lone Ranger started chasing him

h(x) = 49 This is the border's distance from town x hours after the Lone Ranger started chasing the bank robber.

- The graphs show the robber's distance from town and the Lone Ranger's distance from town at each moment after the Lone Ranger started chasing the robber.

- Any vertical distance between ranger's and robber's graphs shows the distance between the two at that moment during the chase.

- The horizontal graph shows how far from town the border is at every moment during the chase.

- The vertical distance between the ranger's and robber's graphs decreases as the number of hours they have run increases, so the Lone Ranger is catching up to the robber.

- However, when the robber hits the border (i.e., is 49 miles from town), the Lone Ranger is less than 49 miles from town. The robber gets away (unless the ranger follows him into Mexico).

Problems

1. A community group has 2000 perimeter-feet of prefabricated wall to build the superstructure for a single-story, rectangular convention hall. The hall needs to be further subdivided by two walls through its interior so that the hall is made of three huge rectangular rooms. What outer dimensions will give the convention hall as much floor space as is possible? (See a GSP sketch of this example and a Graphing Calculator example of an explained model.)

Figure 1

2. Bob drank 2/3L of water in 5/7 minute. In how many minutes will he drink 3/8L of water?

3. An A-frame barn is to be built so that it is 30 ft high, 40 ft wide and 60 ft long. A rectangular room is to be built inside the barn so that its ceiling abuts the roof. What dimensions will maximize the volume of the room?

4. You have a circle of radius 5 ft. Find the dimensions of the triangle circumscribed by this circle that has the largest possible area.

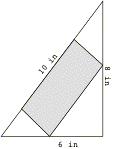
5. A rectangle is to be inscribed in a right triangle having sides of length 6 in, 8 in, and 10 in (Figure 1). Find the dimensions of the rectangle with greatest area assuming the rectangle has two vertices on the triangle's hypotenuse and one vertex on each of the other sides, as in the figure to the right.

6. Jamie Johnson rides frequently with her father to Chicago. On one particular trip it took 2 hours for them to travel the 110 miles from home to Chicago. They made the trip in two parts. Jamie kept an eye on the speedometer and estimated that in the first part they averaged 40 miles per hour. She estimated that in the second part they averaged 60 miles per hour. About how long did they drive in each part of the trip?

7. Statistical data from trucking companies suggests that the operating cost of a certain truck (excluding driver's wages) is 12+x/6 cents per mile when the truck travels at x miles per hour. If the driver earns $6.00 per hour, what is the most economical speed to operate the truck on a 400 mile turnpike where the minimum speed is 40 miles per hour and the maximum speed is 65 miles per hour?