Saturday Math Club
Summary of Lesson from January 3, 1992
and
Assignment for January 10, 1992
The following pages contain a summary of what we did in class on Friday, January 3, 1992. Your assignment for January 10 is to review this summary and reinvestigate each of the items discussed in it. For example, reenact each of the situations described in the summary and come up with alternative explanations to the ones I summarized, or investigate variations of the situations. By next week you should be able to make any of these constructions from scratch and you should be able to explain why each construction works.
There are several places where I inserted notes and
questions for you to think about. Write your responses to these
questions or observations (use Microsoft Word; bring your disk with your
responses on it).
For extra credit" you can write about anything that puzzles you (e.g., Ivonne wondered why it is that every point on a segment's perpendicular bisector is equidistant from the segment's endpoints) and write about your investigations of those situations. I will allot some time during the next lesson to talk about things that puzzle you.
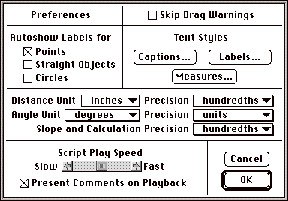
Do this before going through the summary: Start
Sketchpad. Then select Preferences
in the Display menu. If
the box next to Points in the "Autoshow labels" section has an X in it, then just click Cancel. If the box is empty, then click it once, so that it has an X in it. Click OK when your dialog box looks like the figure to the right.
Situation 1: Start
with  .
.
Construct point C, the midpoint of 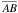 .
.
Move: Point A or
point B.
Question: Why does
point C move the way it does when you move point A or point B?
Our Answer: As you
move point A or point B, the segment changes so that A and B are still its
endpoints. As the segment moves, C must move so that it remains the midpoint of
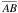 .
.
Situation 2: Start
with 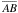 .
.
Construct point C, the midpoint of 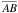 . Construct k, a line
perpendicular to
. Construct k, a line
perpendicular to 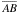 at C.
at C.
Move: Point A or
point B.
Question: Why does
line k move the way it does when you move point A or point B?
Our Answer: There
are two parts to the reason line k moves the way it does. (1) As
you move point A or point B, the segment changes so that A and B are still its
endpoints. Line k must adjust so that it
remains perpendicular to 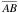 . (2) As the segment moves, C must move so that it remains
the midpoint of
. (2) As the segment moves, C must move so that it remains
the midpoint of 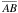 . So, line k
must also adjust so that it still goes through point C.
. So, line k
must also adjust so that it still goes through point C.
Putting (1) and (2) together, as you move point A or point
B, line k moves so that it remains
perpendicular to 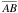 and so that it
passes through the midpoint of
and so that it
passes through the midpoint of 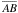 .
.
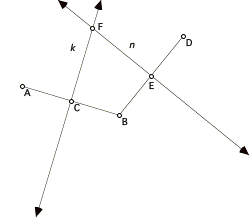
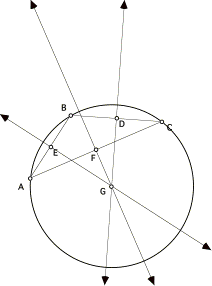
Situation 3: Start
with 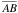 , with midpoint C, and
, with midpoint C, and  , with midpoint E.
, with midpoint E.
Construct line k
perpendicular to 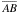 at C. Construct line n perpendicular to
at C. Construct line n perpendicular to 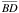 at E. Construct point F, the point of intersection of lines k and n.
at E. Construct point F, the point of intersection of lines k and n.
Move: Point A, point
B, or point D.
Question: Why does
point F move the way it does?
Our Answer: If you
move point A, then line k moves according to the explanation
given in situation 2. As line k moves,
point F must move so that it remains the point of intersection of lines k and n.
If you move point D, then line n also moves according to the explanation given in
situation 2. As line n moves,
point F must move so that it remains the point of intersection of lines k and n.
If you move point B, then both line k and line n must move according to the explanation given in situation 2. As lines k and n
move, point F must move so that it remains the point of intersection of lines k and n.
Situation 4: Start
with 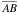 , with midpoint C, and line m perpendicular to
, with midpoint C, and line m perpendicular to  at C.
at C.
Construct point E on m.
Measure the distances between A and E and between B and E.
Move: Point E.
Question: What do
you notice?
Our Answer: Point E
is always equidistant (the same distance) from points A and B.
Conjecture: Any point on the perpendicular bisector of a segment is equidistant from the endpoints of the segment. (This is a conjecture because even though this statement seems to be true, we haven't given any reason why it must be true.)
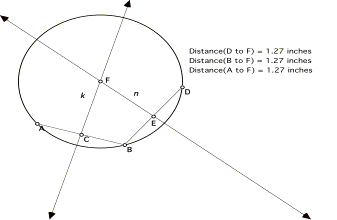
Situation 5: Start
with 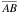 , with midpoint C, and
, with midpoint C, and 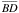 , with midpoint E.
, with midpoint E.
Construct line k
perpendicular to 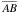 at C. Construct
line n perpendicular to
at C. Construct
line n perpendicular to 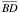 at E. Construct point F, the point of intersection of lines k and n.
at E. Construct point F, the point of intersection of lines k and n.
Construct a circle with F as its center and which passes
through point D (first select point F, then shift-select point D, then choose Circle
by Center + Point in the Construct
menu).
Question: Why does
this circle also go through points A and B?
Our Answer: Point F
is on the perpendicular bisector of 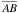 and point F is
on the perpendicular bisector of
and point F is
on the perpendicular bisector of  . According to the conjecture in situation 4 (which we can
only trust tentatively until we give a reason why it must be true), F is
equidistant from A and B, because it is on the perpendicular bisector of
. According to the conjecture in situation 4 (which we can
only trust tentatively until we give a reason why it must be true), F is
equidistant from A and B, because it is on the perpendicular bisector of 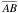 , and F is equidistant from B and D, because it is on the
perpendicular bisector of
, and F is equidistant from B and D, because it is on the
perpendicular bisector of 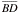 . So, A and B are the same distance from F as is D. But the
distance from F to D was defined to be the length of the radius of the circle.
So the distance from F to B and the distance from F to A are also the length of
the radius of the circle. Therefore, A and B have to be on the circle, since they are just as far from the circle's center as is point D, and we constructed the circle so that it passes through D.
. So, A and B are the same distance from F as is D. But the
distance from F to D was defined to be the length of the radius of the circle.
So the distance from F to B and the distance from F to A are also the length of
the radius of the circle. Therefore, A and B have to be on the circle, since they are just as far from the circle's center as is point D, and we constructed the circle so that it passes through D.
Move: Point A, point
B, or point D.
Question: Why does
the circle always pass through all three of point A, point B, and point D as
you move any of them?
Answer:
Situation 6: Use the
circle tool to make a circle centered at A and passing through B. Put two more
points on the circle. Construct 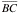 and
and 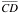 . Construct perpendicular bisectors to
. Construct perpendicular bisectors to  and
and 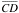
Move: Point A.
Do this: Describe everything that is wrong with this "explanation" of why the circle moves as it does when you move point A.
When you move point A, the lines still have to go through
A, so they have to move to follow A. Also, when the lines move, 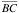 and
and 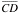 have to move so that the lines are still perpendicular to them and they have to move so that the lines still go through the segment's midpoints. Also, the circle has to go through points B, C, and D, so wherever points B, C, and D move, the circle has to follow them.
have to move so that the lines are still perpendicular to them and they have to move so that the lines still go through the segment's midpoints. Also, the circle has to go through points B, C, and D, so wherever points B, C, and D move, the circle has to follow them.
Situation 7: Construct a circle that passes through all three vertices of ÆABC, so that no matter how you move any vertex of the triangle, the circle continues to pass through all three vertices.
Our Answer: Construct the perpendicular bisectors to each side. Construct G, the perpendicular bisector's point of intersection. Then construct a circle centered at G and passing through any one of the triangle's vertices.
Question: Why doesn't it matter which of the triangle's vertices you use as the "circumference" point when you construct the circle centered at G? (For instance, some people might think
that you can only use G and C to make the circle.)
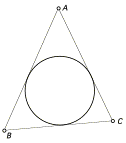
Our goal in this part of the lesson was to develop a construction so that, for any triangle, we can construct a circle that is tangent to each of the triangle's sides (as in the figure to the right). When we construct a circle tangent to each of the triangle's sides, the triangle is called an inscribed triangle.
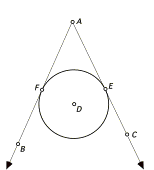
We made these observations about any circle with center D
that is tangent to ÐBAC at point E and at point F (we did not justify these observationsÑthat is something we still need to do):
1) D, the center of the circle, is the same distance from E
as it is from F. The distance between D and E and the distance between D and F
is the radius of the circle. (Ivonne)
2) The segments joining D, the center of the circle with E
and F, the points of tangency, are perpendicular to the sides of the angle. (Micah)
3) D, the center of the circle, is on the angle bisector of ÐBAC. (Daryl)
Observations 1 will help us construct an angle bisector.
Observation 2 will help us construct the circle once we know how to find its
center. Observation 3 will help us locate the center of the circle when we
start with just a triangle.

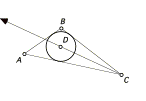
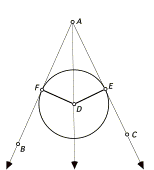
(a) (b) (c)
By observation 3, D, the center of the circle, is on the
angle bisector of ÐBAC (figure a). Also, observation 3 tells us that D, the center of
the circle, is on the angle bisector of ÐACB
(figure b) and is on the angle bisector
of ÐCBA. Thus, we can locate
D, the center of the circle, by finding the point of intersection of the angle
bisectors.
Once we have the center and one point on the circle, we can construct the circle. So, to construct the circle, we can use observation 2Ñwhich tells us that if we construct a line perpendicular to one of the sides through D (which we now can locate by finding the intersection of the angle bisectors), the point of intersection of that side and the line through D perpendicular to it is on the circle.
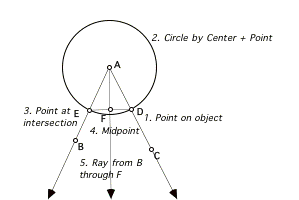
The only thing we needed to figure out by this time was how
to construct an angle bisector. Ivonne suggested this: Label a point on one
side of an angle (using Point on Object).
Construct a circle centered at the vertex and passing through that point. Find
the intersection of the circle with the other side of the angle. We now have
two points that are the same distance form the vertex of the angle. The point
that is half-way between them is on the angle bisector, so bisect the segment
connecting these two points. The line containing the midpoint of the segment
and the vertex of the angle is the angle bisector. (Now that we have figured
out how to bisect an angle, we can use the Sketchpad command Angle
Bisector in the Construction
menu.)
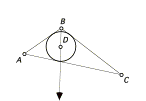
Now we can construct the circle that is tangent to each side
of a triangle. The figure to the right shows the end-product of the
construction. (I hid the stuff made when bisecting each of the angles.)